Basic HTML Version
38
Geotechnical News • December 2012
www.geotechnicalnews.com
GROUNDWATER
errors once a finite element solution
has been obtained. The procedure
is referred to “adaptive” since the
process depends on previous results at
all stages.
Various procedures exist for the
refinement of finite element solutions.
Broadly these fall into two categories
(Zienkiewicz et al., 2005).
1. The
h
-refinement in which the same
class of element continues to be
used but it is changed in size, in
some locations while being made
larger in some locations and small-
er in others, to provide maximum
economy in reaching the desired
solution,
2. The
p
-refinement in which the same
element size is used and there is a
simple increase, generally hierar-
chically, in the order of polynomial
used in the definition of the ele-
ments.
It is occasionally useful to divide the
above categories into subclasses, as
the
h-
refinement can be applied and
thought of in different ways. Three
typical methods of
h
-refinement are:
1. Element subdivision – if existing el-
ement show too large an estimated
error, the elements are simply di-
vided into smaller elements while
keeping the original element geom-
etry boundaries intact,
2. Mesh regeneration (remeshing) – on
the basis of a given solution, a new
element size is predicted in all the
domains and a totally new mesh is
generated,
3. r
-refinement – keeps the total num-
ber of nodes constant and adjusts
their position to obtain an optimal
approximation. This method is dif-
ficult to use in practice and there is
little reason to recommend its us-
age.
The
p
-refinement subclasses are:
1. one in which the polynomial order
is increased uniformly throughout
the entire domain,
2. one in which the polynomial order
is increased locally while using hi-
erarchical refinement.
Occasionally it is efficient to combine
the
h
- and
p
- refinements and call it
the
hp
- refinement. In this procedure
both the element size and the polyno-
mial degree,
p
is altered.
Advantages of using automatic
adaptive mesh generators
(numerical examples)
Advantages of using automatic
adaptive mesh generators are illus-
trated through comparison of results
obtained on the numerical models
analyzed by Chapuis (2012). Chapuis
(2012) presented two examples prob-
lems where he created finite element
meshes semi-automatically and solved
the seepage problems. The same
example problems were solved using
automatic mesh refinement using the
SVFlux / FlexPDE finite element
code.
Cut-off example
The geometry of the model (i.e., dam
with partial cut-off wall; k
sat
,
homogenous
soil
= 8.13×10
-3
m/day) analyzed is
presented in Figure 1.
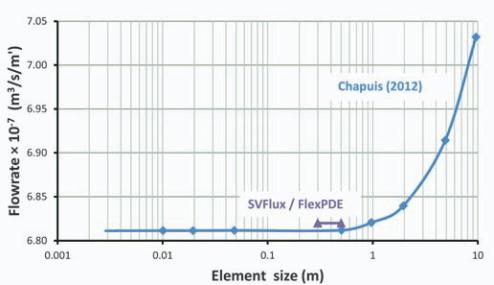
In the reference article, convergence
of the solution was obtained using a
uniform mesh with an element size of
0.5 m. From Figure 1 it can be seen
that the converged solution obtained
when using the automatic adaptive
mesh refinement has larger elements
in most parts of the analyzed domain.
The exception is found around the
cut-off wall where the element size is
significantly smaller than the overall
average element size. For the mesh
presented in Figure 1, the calcu-
lated flow rate was 6.82×10
-7
m
3
/s.
Calculation time for the mesh pre-
sented at Figure 1 was 0.01 minutes.
Comparison of results obtained with
manually-controlled meshes and
automatic-controlled adaptive meshes
are presented in Figure 2. Calculation
computational times associated with
using a disabled mesh generator with
a specified maximum element size of
0.5 m, increased to 7.37 minutes while
the flow rate solution remained the
same (note that an older computer was
used for this study). The consequence
of further reductions in the element
size to 0.3 m was an increased calcula-
tion time from 7.37 minutes to 36.03
Figure 1. Partial cut-off wall model geometry with mesh
generated using the automatic adaptive mesh generator.
Figure 2. Converged leakage flow-rate for the cut-off
example.

